Yesterday, a friend of mine who defines himself as a creation-believing-Christian suggested that I attend a lecture titled Creation: The Missing Link. I couldn't resist replying with a tirade against Creationism. Here it is.
The results in Molecular Biology have demonstrated beyond any reasonable doubt that evolution is sufficient to explain the variety of species that exist (or have gone extinct). Last December, I read a very detailed and documented book that demonstrates just that: The Making of the Fittest - DNA and the ultimate forensic record of evolution, by Sean B. Carroll ().
Faith and Science are two different things. Creationism and Intelligent Design can explain everything. I know. But the best proofs they can count on are tautological.
Peer-reviewed published Science is not infallible, but when the body of evidence grows over decades and no measure, experiment, or discovery invalidates a theory, the probability that the theory is false vanishes. Relativity, for example, is a theory and, as such, it could be refined in future, exactly as Relativity itself refined Newtonian Mechanics. But, after so many confirmations, it cannot ever be invalidated, exactly as Newtons theories have not been.
The Global Positioning System only works because the clocks on the satellites are adjusted to take into account Relativity. The Cosmological Constant introduced and then repudiated by Einstein to avoid divergence of his formulae has been re-introduced to be able to avoid contradiction with the fact that the universe is expanding at an accelerated rate. Dark Energy and the Cosmological Constant are ad-hoc solutions to explain (without actually explaining anything) the expansion of the universe. This is a clear indication the Relativity as we know it is part of a more general theory that will explain the universe without fudging the formulae. But that doesn't invalidate Relativity at all.
All theories go through successive refinements and generalisations.
Another "fudge" in Science that I know of is the "renormalisation" used in Quantum Field Theory to get rid of infinities (a bit like saying that 0/0 = 1!). Again, it means that the theory will probably be refined in future (unless it becomes a particular case of a more general theory that doesn't need renormalisation). Meanwhile, Quantum Theory has explained to us semiconductors, superconductivity, superfluidity, and a host of other useful stuff.
Evolution is yet another theory and, like Newtonian Mechanics, Relativity, and Quantum Mechanics, will probably be refined in future. But meanwhile, it explains the diversity of Nature without need for any external intervention. And the aim of any explanation must be to be parsimonious: don't do anything more than necessary to achieve a certain result. The fewer the ad-hoc factors invoked to explain something, the better (Occam's Razor).
This does not (and I don't see why it should) prevent you from believing that the laws of Physics are what they are because Somebody invented them and then set the universal constants exactly to the value they currently have. But, as I said, it is then a matter of Faith, not of Science.
Sometimes (but very very very seldom! ;-) I wish I believed in God. I know it can be of great help. But I don't believe, and don't think I ever will (although, obviously, the future cannot be really predicted...)
My wife is reading the book "Religion for Atheists", written by the Philosopher Alain De Botton. You can see an interview with him on the 7.30 Report's website. She loves it, and I will also read it. Although he is a staunched Atheist, he claims that we should respect Religion and try to learn from it, rather than dismiss it. He says that Religion does certain thing very well and that we miss some of them in our modern secular world. I just watched the interview on the web and it is absolutely worth watching. I strongly encourage you to do so. Alain is not just brilliant and articulate. He is also caring. I can tell you this: if everybody thought like him, religious or atheist, I doubt that there would be any religious persecution in this world.
I use this blog as a soap box to preach (ahem... to talk :-) about subjects that interest me.
Tuesday, February 28, 2012
Sunday, February 26, 2012
Board Games
Years ago, I played some board games like Monopoly, Diplomacy, and Halma. But then, for whatever reason, I stopped. Earlier this year, I was talking with Glen of Mind Games about numeric puzzles and he suggested that I attend Cancon 2012, Canberra's biggest gaming convention, organised by the Canberra Games Society.
I was there all three days and looked at several games, like Carcassonne, Dominion, Tanto Cuore, and Alien Frontiers.
I discovered that:
Anyhow, the visit to the Convention made me itchy. I wanted to buy a game and play it. But they are so expensive, and none of them was completely right for me. And my wife, who was going to be my most regular adversary, is even more choosy then me...
I ended up not buying any game and deciding to develop one. For one thing, I didn't want to have to learn thick manuals before being able to play.
My first attempt was an abstract game named "Beeing About".
You start with the following 112 tiles that you lay on a board.

The board consists of 169 hexagonal cells and 48 half-cell edges, arranged to form a large hexagon. Half of the edges are marked to 'connect' the two adjacent cells. Seven of the cells are 'crossroads' marked in such a way that all six sides are connected with each other:
The game preparation is trivial: Each player draws a number of tiles from the bag and places them face up on the table, where they are visible to all players. The number of tiles drawn by each player is 6 with two players, 5 with three or four players, and 4 with five of six players.
The whole rule book is as follows.
Each player in turn plays a tile by laying it on one of the hexagons of the board. For a play to be valid, the following rules must be respected:
And here are examples of incorrectly laid tiles, because not all adjacent paths connect:
After laying a tile, a player draws a new tile from the bag, unless the bag has been emptied.
If a player cannot lay any of his tiles, he can replace one or more of his tiles with tiles from the bag. To do so, he places his tiles into the bag and gives it a good shake before drawing from it the same number of tiles. This obviously means that, especially towards the end of the game, he could draw the same tiles he has just discarded. If, after replacing tiles, the player still cannot play any of them, he sits his turn. That is, a player can only exchange tiles once before each one of his turns, and only if he cannot play any of the tiles he is holding.
Instead of exchanging tiles, a player can also decide to sit a turn. If the bag is empty, the player obviously cannot exchange tiles and is forced to sit the turn.
A tile can be laid on a crossroad cell, but only if its paths connect to the paths of all tiles adjacent to the crossroad.
That's it.
I played it a few times, and it turned out that strategising was not really possible, because the players couldn't plan beyond their second next move. In Carcassonne, you draw a tile at a time, but the mix of tiles and their meaning make possible for you to roughly plan some moves ahead. Carcassonne is a tile-laying game with very simple rules and very smartly designed. That's why it is so successful.
I could have worked on "Beeing About" and improve its strategising possibilities, but the game had another problem: it was too abstract. The most successful new board games don't only tickle your intellect. They also stimulate your imagination through nice sceneries and dazzling graphics. And I suddenly had the urge to develop a successful board game.
For the graphics, I will have to find a partner, but I feel that "Beeing About" is not so suitable anyway. I am now working on a different game, based in space. More about that in a future article. For now, I shall only say that it also has a board of hexagonal cells. Hexagons are much more exciting than squares or triangles. Don't you agree?
Before I end this article, here are some further reflections on the tiles of "Beeing About".
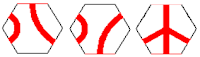
I wanted to draw all possible combinations of connections between the sides of hexagons. The tiles with 1 point of contact (the black dead end) and with 2 (green) and 3 (blue) points do just that. But if you carefully look at the tiles with 4 points of contact (red), you will notice that several of them are redundant. In the game, the only thing that matters is the distribution of points of contacts on the edge of the hexagons. How they are connected within the tile is irrelevant. For example, the following three tiles:
have the same points of contact among themselves. And the following ones too:
and these:
Only three combinations of four points of contacts are functionally distinct:
It makes sense, because having four points of contacts means that you leave two of the six sides free. And we know from the tiles with green paths that there are only three ways in which you can choose two sides...
Similarly, the tiles with five points of contacts (magenta) are all functionally identical, because there is only one way of leaving a side of the hexagon free of contacts.
But the different patterns looked nice...
I was there all three days and looked at several games, like Carcassonne, Dominion, Tanto Cuore, and Alien Frontiers.
I discovered that:
- Some games, like Tanto Cuore and Alien Frontiers have very complex rules. Certainly, they create an almost unlimited number of different situations. But they also make it necessary to play quite a bit before becoming proficient and exploiting all possibilities. Too many things to remember, especially for an impatient person like me.
- All games have an element of randomness, realised by drawing cards, rolling dice, or picking tiles.
- All games make possible to develop strategies to improve your chances of winning. Sometimes it is realised by letting the players buy other cards (like in Dominion and Tanto Cuore). Or by choosing between different playing options (like in Alien Frontiers), or deciding where to lay a tile (like in Carcassonne).
- Most games hev extensions. Their purpose clearly is twofold: keep the game new and stimulating, and make more money for the game developer and publisher!
Anyhow, the visit to the Convention made me itchy. I wanted to buy a game and play it. But they are so expensive, and none of them was completely right for me. And my wife, who was going to be my most regular adversary, is even more choosy then me...
I ended up not buying any game and deciding to develop one. For one thing, I didn't want to have to learn thick manuals before being able to play.
My first attempt was an abstract game named "Beeing About".
You start with the following 112 tiles that you lay on a board.

The board consists of 169 hexagonal cells and 48 half-cell edges, arranged to form a large hexagon. Half of the edges are marked to 'connect' the two adjacent cells. Seven of the cells are 'crossroads' marked in such a way that all six sides are connected with each other:
The game preparation is trivial: Each player draws a number of tiles from the bag and places them face up on the table, where they are visible to all players. The number of tiles drawn by each player is 6 with two players, 5 with three or four players, and 4 with five of six players.
The whole rule book is as follows.
Each player in turn plays a tile by laying it on one of the hexagons of the board. For a play to be valid, the following rules must be respected:
- The played tile must be in contact with at least one of the tiles that are already on the board. Alternatively, the tile can be laid adjacent to a crossroad cell, but only if the crossroad is already in contact with one or more tiles. The only exception to this rule is when the first player plays his very first tile. In that case, he lays the tile in contact with the crossroad in the middle of the board.
- The paths of the played tile must continue the paths of the tiles (and possibly of a crossroad or an edge) to which it comes into contact. Note that the colours of the paths are different only to easily distinguish the tiles with different numbers of contact points.
And here are examples of incorrectly laid tiles, because not all adjacent paths connect:
After laying a tile, a player draws a new tile from the bag, unless the bag has been emptied.
If a player cannot lay any of his tiles, he can replace one or more of his tiles with tiles from the bag. To do so, he places his tiles into the bag and gives it a good shake before drawing from it the same number of tiles. This obviously means that, especially towards the end of the game, he could draw the same tiles he has just discarded. If, after replacing tiles, the player still cannot play any of them, he sits his turn. That is, a player can only exchange tiles once before each one of his turns, and only if he cannot play any of the tiles he is holding.
Instead of exchanging tiles, a player can also decide to sit a turn. If the bag is empty, the player obviously cannot exchange tiles and is forced to sit the turn.
A tile can be laid on a crossroad cell, but only if its paths connect to the paths of all tiles adjacent to the crossroad.
That's it.
I played it a few times, and it turned out that strategising was not really possible, because the players couldn't plan beyond their second next move. In Carcassonne, you draw a tile at a time, but the mix of tiles and their meaning make possible for you to roughly plan some moves ahead. Carcassonne is a tile-laying game with very simple rules and very smartly designed. That's why it is so successful.
I could have worked on "Beeing About" and improve its strategising possibilities, but the game had another problem: it was too abstract. The most successful new board games don't only tickle your intellect. They also stimulate your imagination through nice sceneries and dazzling graphics. And I suddenly had the urge to develop a successful board game.
For the graphics, I will have to find a partner, but I feel that "Beeing About" is not so suitable anyway. I am now working on a different game, based in space. More about that in a future article. For now, I shall only say that it also has a board of hexagonal cells. Hexagons are much more exciting than squares or triangles. Don't you agree?
Before I end this article, here are some further reflections on the tiles of "Beeing About".
I wanted to draw all possible combinations of connections between the sides of hexagons. The tiles with 1 point of contact (the black dead end) and with 2 (green) and 3 (blue) points do just that. But if you carefully look at the tiles with 4 points of contact (red), you will notice that several of them are redundant. In the game, the only thing that matters is the distribution of points of contacts on the edge of the hexagons. How they are connected within the tile is irrelevant. For example, the following three tiles:
have the same points of contact among themselves. And the following ones too:
and these:
Only three combinations of four points of contacts are functionally distinct:
It makes sense, because having four points of contacts means that you leave two of the six sides free. And we know from the tiles with green paths that there are only three ways in which you can choose two sides...
Similarly, the tiles with five points of contacts (magenta) are all functionally identical, because there is only one way of leaving a side of the hexagon free of contacts.
But the different patterns looked nice...
Sunday, February 5, 2012
Banks!
This morning, I saw an interesting advertisement in the window of a bank. It offered an interest rate of 5.6% for a three-month term deposit (yes. In Australia we have high interest rates. This is one of the reasons why people pump up the value of the AUD by investing in it). Then, on the same ad, with the figures well aligned, it offered to pay 5.10% for a six-month term deposit.
Do you get it?
5.6%
5.10%
They expect the interest rates to decrease. Therefore, they offer a lower interest rate for six months than for three months. But, by writing 5.10 instead of 5.1, they make it look as if the interest rate for six months were higher than that for three months. They play on the ignorance of people who see 10 greater than 6 even if it is after a decimal period.
I hate banks... Almost as much as insurances...
Do you get it?
5.6%
5.10%
They expect the interest rates to decrease. Therefore, they offer a lower interest rate for six months than for three months. But, by writing 5.10 instead of 5.1, they make it look as if the interest rate for six months were higher than that for three months. They play on the ignorance of people who see 10 greater than 6 even if it is after a decimal period.
I hate banks... Almost as much as insurances...
Subscribe to:
Posts (Atom)